R.1412
Track behaviour
A railway bridge may support a load of several hundred tons in mid air. The load is transmitted through various parts of the structure to the foundations, where it dissipates into the ground. You might not think of the track itself as a ‘structure’ but in fact it does a similar job on a smaller scale. Here, the structure consists of three main elements: rails, sleepers and road bed. They work together to support the wheels of a moving train, and spread out the load so the ground underneath doesn’t give way. Their behaviour determines the maximum weight per axle of the train and the speed at which it can travel. The engineer’s task is to work out the cheapest possible configuration that meets two requirements. First, the rails mustn’t break, and second, any deflection under the wheels of a train should fall within an acceptable range: too much and a train may jump the rails, too little and the track will provide a rough ride and wear out prematurely.
Effect of static loading
A wheel can exert a force in any of several different directions on the rail, but here we are interested mainly in the vertical load. On a freight line, a wheel load can be as much as 20 tonnes, [21], but on the passenger network in Europe, it is rather less: the maximum permitted values are set out in table 1. For mainline traffic the greatest load per axle (two wheels together) is 22.5 tonnes, roughly twice the equivalent for a heavy lorry. To estimate the impact of wheel loads on the track, we need first to consider what happens when the train is standing still.
| Rail system | Vehicle | Axle load | Wheel load |
|---|---|---|---|
| (tonnes) | (tonnes) | ||
| Mainline | 17.0 | 8.5 | |
| Mainline | Other | 22.5 | 11.25 |
| Metro | 10.0 | 5.0 | |
| Tram | 6.0 | 3.0 |
The Winkler beam model
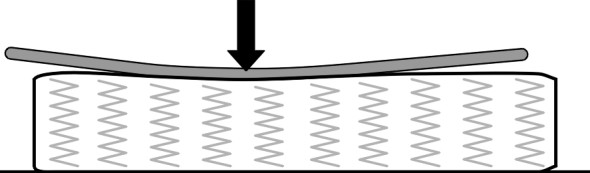
The track is a complex structure. Several methods have been devised to predict the stresses and strains within each of its components (see for example [26]), of which the most sophisticated involve computer simulation. But much can be learned by visualising the rail as a simple beam that rests on a springy surface, rather like a broomstick resting on a mattress. If you press down in the middle of the broomstick it will bend, sagging in the centre and compressing the mattress (figure 1). As the mattress deflects it provides increased resistance, and the reaction that supports the broomstick varies along the length of the broomstick in proportion to the deflection at each point. Expressed in mathematical terms, this idealised structure is known as a Winkler beam, after the German pioneer of structural theory Emil Winkler (1835 - 1888), who first conceived the ‘elastic foundation’ as a tool for analysing and explaining the interaction between structures and the ground on which they stand. When applied in a railway context, the broomstick represents the rail while the mattress represents the sleepers and roadbed as if they formed a continuous body that flexes slightly under load. Although in reality the sleepers are discrete objects, the model yields quite good predictions of the deflection at different points along the rail, and it uses only three numerical parameters: Young’s modulus \(E\) for the steel rail, the moment of inertia \(I\) for the rail cross-section [39], and a stiffness parameter \(k\) that represents the force per unit length required to produce unit deflection of the roadbed.
Figure 1
The details can be found in a classic work on structural engineering [40] and specifically in a railway context in [7] and [27]. Each uses a different notation and we shall largely follow [7]. Without trying to prove them, we will set out here the most important formulae that describe the behaviour of the track in response to a single wheel load, and use them to map out the deflections and stresses in the rail nearby. For a wheel considered as a point load \(P\), the deflection \(w(x)\) of the track at a point located at a distance \(x\) downstream is the product of a constant term \(P/2kL\) and a dimensionless ‘shape function’ \(\eta (x)\) thus:
(1)
\[\begin{equation} w(x) \quad = \quad \frac{P}{2kL} \eta (x) \end{equation}\]where \(L\) is the characteristic length given by
(2)
\[\begin{equation} L \quad = \quad \left( \frac{4EI}{k} \right)^\frac{1}{4} \end{equation}\]and the shape function by
(3)
\[\begin{equation} \eta (x) \quad = \quad e^{- x/L} \left[ \cos \left( \frac{x}{L} \right) \; + \; \sin \left( \frac{x}{L} \right) \right] \end{equation}\]The result is a sine curve of wavelength \(2 \pi L\) whose amplitude decays with distance away from the origin. The greatest deflection occurs immediately under the wheel at \(x = 0\). Here, the shape function takes on the value 1, and the deflection is given by
(4)
\[\begin{equation} w_{\max } \quad = \quad w(0) \quad = \quad \frac{P}{2kL} \end{equation}\]A typical profile is shown in figure 2 for a wheel load of 125 kN on a track with a good quality roadbed and a stiff rail (\(k = 0.09\) kN mm\(^{-2}\) and \(L = 700\) mm). If you just look at the right hand part of the curve, you may find the shape familiar. It is mathematically identical to the deflection profile traced out by a car bouncing freely on its suspension after a sudden impact as shown in the upper diagram of Figure 5 in Section G1115, except of course that the deflections of the car body vary over time, whereas the deflections in the rail vary over distance. Both are damped, and the motion decays rapidly from one cycle to the next. Figure 2 shows that the maximum deflection of the rail is around 1 mm, and also that the deflection is negative a little distance away on either side, where the rail actually rises above its normal level. The model as presented here does not take into account the weight of the track, which presents an additional load to be superimposed on the system. While the weight of modern concrete sleepers is usually enough to prevent them from lifting clear of the ground [9], nevertheless, the rail surface does in fact rise. It seems that the wheel drags along with it a depression or valley in the track together with a small hill fore and aft.
Figure 2

So much for the deflections, but what about the stresses? First, we must determine the bending moment in the rail, which is related directly to the profile of deflections. For readers not familiar with structural analysis, we obtain the bending moment at any point in a beam by cutting the beam at that point in our imagination. Let’s assume the beam is horizontal. Then ignoring the left-hand piece we work out the couple exerted at the severed cross-section by the various loads and reactions acting on the right-hand piece. As a check, we can then take the left-hand piece and work out the couple acting at the severed cross-section in the same way. Since the beam is in equilibrium, the two couples should be equal, and opposite in sign. The convention among structural engineers is to register a sagging moment as negative and a hogging moment (which produces an upward bulge) as positive. For the Winkler beam model, it can be shown that the value of the bending moment is given by the expression
(5)
\[\begin{equation} M(x) \quad = \quad - \frac{PL}{4} \mu (x) \end{equation}\]where the shape function \(\mu (x)\) is given by
(6)
\[\begin{equation} \mu (x) \quad = \quad e^{- x / L} \left[ \cos \left( \frac{x}{L} \right) \; - \; \sin \left( \frac{x}{L} \right) \right] \end{equation}\]Figure 3
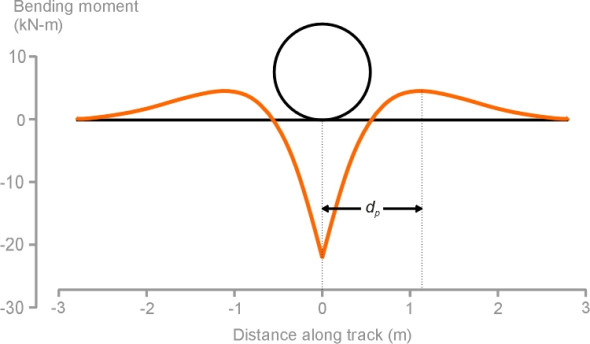
figure 3 shows the shape of the curve given the parameters specified earlier. The bending moment is negative in the neighbourhood of the wheel, indicating a sagging mode as one might expect. On either side it rises to a positive value that can be shown to peak at a distance \(d_{p}\) given by \(\pi L/2\) which in this case comes to a little over a metre [8]. This is an important point and we’ll return to it later. The largest numerical value of the bending moment is negative in sign and it occurs directly under the wheel at \(x = 0\), where the shape function \(\mu(0) = 1\). Its value is given by
(7)
\[\begin{equation} M_{\min } \quad = \quad M(0) \quad = \quad - \frac{PL}{4} \end{equation}\]Stresses and strains
The first requirement of a railway track is that it shouldn’t break. Nor should it distort in such a way as to derail the train. Let’s look at the possibility of a broken rail first. Using a model such as the Winkler beam, or a computer simulation package, one can estimate the stresses in the rail that arise from bending under a wheel load in the vertical plane. As we saw earlier in Section R1605, the stress induced by the bending moment varies over the rail cross-section. The maximum compressive stress occurs at the upper surface, and the maximum tensile value at the lower surface (figure 4). Mathematically, we picture this as a negative stress at the top of the rail head that changes linearly to a positive value at the foot, passing through zero at the neutral axis near the centre. For the above example with UIC 54 rail, the peak stresses turn out to be nearly -90 MPa at the top and nearly 80 MPa at the bottom.
Figure 4
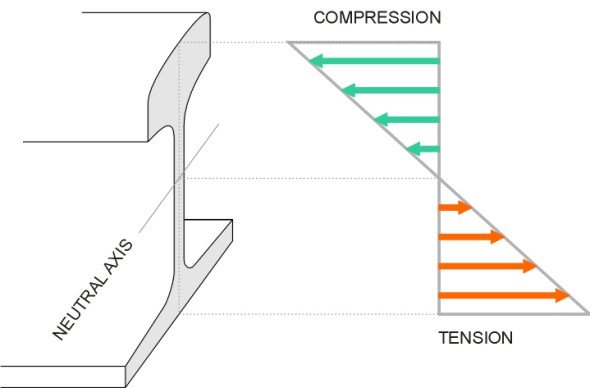
Figure 5
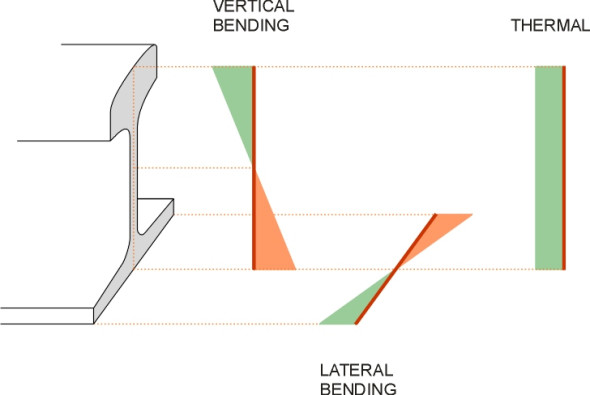
To the bending stresses must be added the thermal stresses in the rail: tensile in cold weather when the rail tends to shrink, and compressive in hot weather when it expands. Both act more-or-less uniformly over the whole cross-section. And on a curve, the train wheels push the outside rail sideways so that it bends in the horizontal plane, creating extra tension on the outside edge and compression on the inside. If we superimpose these on the bending stresses as shown schematically in figure 5, we can then judge whether the total at any point in the cross-section exceeds the allowable stress for the rail material used. Sample calculations are set out in [10]. So far, we have ignored the additional loads associated with the impact of the wheel on irregularities in the rail alignment, which we’ll look into later. For the moment, it’s worth making two general points.
Figure 6
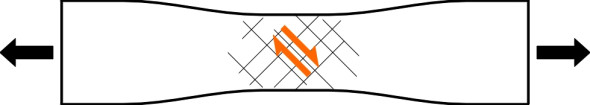
The first is about the failure of the rail. How does a rail break, and what are the possible causes? The wheel load produces a large vertical stress within the contact patch. This is complemented by the longitudinal stresses caused by bending and thermal loads. But none of these stresses will cause a metal to yield or crack, at least not directly. Metals don’t fail in compression or tension as such. But a tensile or compressive force will set up a shear stress within the material, and it is in the plane of maximum shear stress, often around \(45^\circ\) to the applied load, that the failure occurs, because the atoms slide over one another more easily than they pull apart (figure 6). But there’s another factor at work. If there were no longitudinal stresses, the loading pattern would be symmetrical with a vertical contact stress only, and any plane at \(45^\circ\) to the vertical contact stress would serve as a plane of failure. But in the case of a railway track, the longitudinal stresses destroy the symmetry, effectively reducing the shear in the \(xz\) plane and leaving the shear in the lateral \(yz\) plane dominant [6]. Hence we would expect the material to yield and crack laterally across the rail from side to side rather than in a longitudinal direction, which seems to agree with the predominant fatigue cracking modes referred to in Section R2019. This doesn’t mean that the bending stresses are benign. With the passage of each wheel, the material goes through a loading cycle, and it is the rise and fall that puts energy into the rail, encouraging cracks to propagate and potentially leading to failure over time.
Figure 7
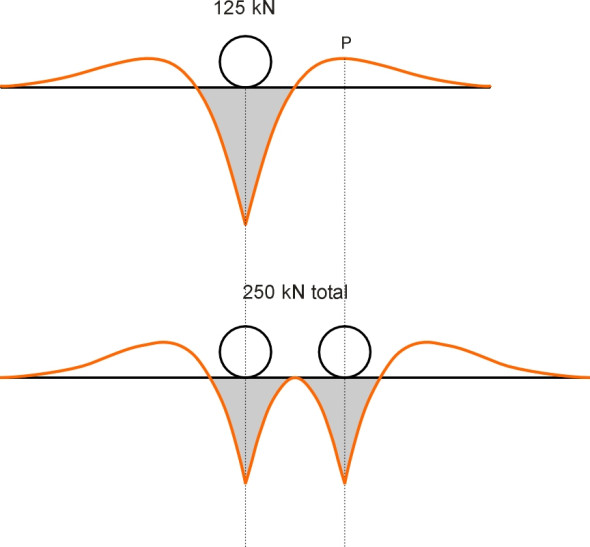
The second point is related to the first because it, too, is concerned with bending moment reversals. But here we are dealing with the bending moment profile in space rather than time. So we must step back and view what happens along an appreciable length of track, and in particular, the way in which the bending moment switches from negative to positive, peaking at a distance \(d_{p}\) on either size of the wheel load (figure 3). The point is that no railway vehicle can balance on a single axle - there must be at least two. If we can choose the spacing between axles, where do we put the second one? A sensible place might be at the point P of the profile shown in the upper part of figure 7. If we add a second wheel load here of equal magnitude to the first, the profile of bending moment along the rail changes. To find it, we simply add the profile for the original wheel and the profile for the second wheel, which is shifted along the rail by a distance \(d_{p}\). The trough in the first wheel load is partly cancelled by one of the peaks in the first, and vice versa, so that overall, the maximum stresses are reduced. You can see in the lower part of figure 7 that with the additional wheel load the size of the bending moment ‘trough’ under each wheel is significantly reduced. It’s worth pausing to take in the significance of what is going on here. It may seem paradoxical at first sight, but we can double the load at the same time as relieving the stress in the rail - a case of adding more weight to ease the burden as it were. Or to put it another way, we can take a twin-axle freight wagon and convert it into a four-axle bogie vehicle and thereby reduce the rail stresses by more than a half. If the Winkler beam theory is accurate, then the optimum bogie wheelbase will be \(\pi L/2\). With modern track, whose characteristic length L is typically less than 1 metre, the optimum works out at 1.5 m. In practice, a modern bogie needs a longer wheelbase of around 2.5 m for safe running at high speeds, so the benefit is not as large as it might be.
Now for the deflections. In the past, rails used to move up and down quite a lot, and if you stood by a level crossing gate and watched what happened, you would have noticed them sink then rise again under the weight of each wheel. During the steam era, the deflection at a rail joint could be more than 10 mm [41] but with modern continuously welded rail it is barely noticeable: typically around 2 mm, and less for concrete slab track [30]. Thinking about the deflections leads one to question how ‘stiff’ the track should be. A degree of flexibility helps to reduce the dynamic impacts, but we don’t want the rail to deflect more than a millimetre or two on a high-speed track, especially on a curve or a switch where there is a real possibility of derailing the train. If we want to increase the stiffness, there are two ways of doing it: (a) make the roadbed deeper, or (b) use a heavier rail. Which works best? Here again, the Winkler beam model can help. It turns out that a one percent increase in the roadbed stiffness parameter \(k\) reduces the deflection by about 0.75%. An alternative is to stiffen the rail itself by choosing one with a larger cross-section, but it seems that a 1% increase in area produces a more modest fall in deflection of 0.5%. On the other hand, a heavier rail helps to relieve the bending stresses. Further details about the effects of varying each of the parameters referred to earlier on rail stress and deflection are set out in the Appendix at the end of this Section.
Dynamic behaviour
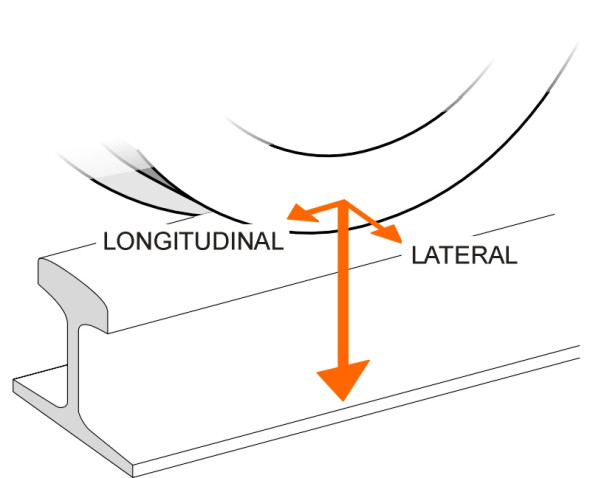
So what happens when the train moves? The depression or valley in the rail is carried along with the wheel, and provided the track is straight and the train doesn’t go too fast, the bending moment profile and the profile of stresses in the track are carried along too, almost unchanged. But on a curve, the inertia of the vehicle comes into play. The vehicle undergoes centripetal forces acting on the outside wheels, which constrain its motion, pushing it sideways towards the centre of curvature. From the point of view of the rail, the outside wheels react laterally outwards against the railhead which adds a horizontal component to the wheel load (figure 8). There is also a transfer of vertical load from the inner wheels to the outer wheels. In principle, for a curve of constant radius one can work out these extra loads and treat them as static forces that don’t change while the vehicle is travelling round the curve. Less easy to deal with are the irregularities in the track alignment - both on curved and on straight track - that create shock loads that can’t be predicted in terms of track geometry.
Figure 8
Shock loads
The rail surface is not as smooth as one might think. On older tracks, there are gaps at the fishplates where the rail sections are bolted together, and each gap delivers a jolt to the suspension of a moving train. Even a continuously welded rail will produce shock loads at the joints. When heated during the welding process, the surface will deform slightly on either side of each weld, so the rails meet at a shallow angle rather than a straight line. There may also be a slight discontinuity if the butt ends aren’t perfectly aligned. So the joints can be a problem, but what about the rail itself? The rail is never perfectly smooth because over time, particles flake away from the surface, and material is gouged out by loose particles and grit from the ballast that gets caught between the wheel and the rail. Since there are no rubber tyres, each bump injects a disturbance into the vehicle suspension: that’s why you can feel the wheels rumbling along the rails when you ride in a tram. People who live close to a railway feel it too because the process works both ways. The wheels generate vibrations that propagate along the rails and in turn radiate noise into the surrounding environment. Vibrations are propagated through the ground to buildings nearby and along the track ahead of the train (which leads to the interesting question, which we’ll come to later, of what happens if a train catches up with its own waves).
Let’s stay with the wheel tread for the moment. When the wheel tread encounters small irregularities in the rail surface, the reaction between the wheel and the rail varies over a considerable range on either side of the static load. The difference between the static load and the dynamic load is rather like the difference between the weight of a milk bottle resting on a table, and the force it generates momentarily when you knock it over. How should one extrapolate from the one to the other? In practice, engineers do what they often do in such circumstances: they assume the two are in direct proportion and express the dynamic element through an empirical factor, a multiplier which in this case is not a fixed ratio for all circumstances - its value depends on vehicle speed. For conventional track the maximum dynamic load is taken as about 50% greater than the static wheel load, so the multiplication factor is 1.5. Experience with the TGV system operating at 300 km/h suggests a ‘dynamic impact factor’ \(\eta\) of about 2.0, meaning that the maximum stresses are about twice the static ones for a vehicle moving on high-quality track at that speed [28].
Such factors can be incorporated into Winkler-type formulae and used to predict dynamic forces on the sleepers and track. But a more ‘scientific’ way to get to grips with shock impacts is to represent the various elements of the track as springs, masses and dampers in the same way as one might represent a vehicle suspension. Because there are so many interacting elements, it isn’t possible to work out explicit formulae for the track response, and the problem must be solved numerically using computer software.
Track deterioration
So what are the consequences of a rough rail surface from the railway operator’s point of view? They are worse perhaps than you might expect. Whenever the rail jolts the wheel, the wheel reciprocates by jolting the rail, which increases the rate of wear and tear on the track as a whole. The wear and tear results in more irregularities that in turn exacerbate the shock loads - it is a circular process, or if you like, a spiral of deterioration. A smooth track will stay smooth for a considerable time while a rough track will get worse at an accelerating rate [12]. That’s why modern high-speed track is aligned very precisely and irregularities kept within narrow limits through intensive maintenance. High-precision track requires (a) a smooth and uniform ballast layer profile and (b) straight rails with a smooth running surface, all at wavelengths ranging from a few centimeters to many metres. At the longest wavelengths, an error of 1 or 2 mm can have significant effects, while at the shortest wavelengths, microns matter [13].
While irregularities in the vertical profile are the ones that most concern railway engineers, irregularities in the horizontal profile matter too. The Tokaido Shinkansen railway provides a good example. Before the line was opened, a high-speed test was carried out with a 4-car electric train at 240 km/h. During the test, one car developed a zig-zag ‘hunting’ motion, and in subsequent runs, other cars began to hunt at the same point along the track. It turned out that the track had deformed laterally, ‘snaking’ from side to side, and the engineers concluded that one particular bogie in poor condition had caused the problem by exerting a lateral force of about 10 tonnes at intervals along the track. The displaced track then triggered hunting in the other bogies. After the service was launched, the railway operator decided to measure the vibrational acceleration daily, and where necessary, to adjust the track overnight [42].
Frequent re-alignment is of course expensive, and in the case of a conventional ballasted track, it’s not very efficient, because as we saw in Section R1602, the ballast is relatively unstable for some time after tamping. It may even have a ‘memory’ of its former state [11] and will settle towards the irregular profile that the adjustment was intended to correct. It has been suggested that for this reason, ‘rough’ or poor sections of track deteriorate more quickly after treatment than smooth sections, not because of any underlying weakness in the subgrade but because the track inherits irregularities that have been worsened by dynamic wheel loads early in the life of the track.
Figure 9

Noise and vibration
Passengers waiting on a station platform know when a train is coming because vibrations propagate along the rails for some distance ahead. The track ‘sings’. The train sings too, because as we saw in Section R1610 each wheel shares many of the characteristics of a church bell.
The resonating track
If the wheel is a church bell, the rail is a tuning fork, or perhaps more aptly, a xylophone: like the bars of a xylophone, successive segments of rail can vibrate between their supports (figure 9). When stimulated by a train wheel rolling over small imperfections in the rail surface, each resonates with a natural frequency \(f_N\) whose value depends on the distance \(S\) between its supporting sleepers together with the mass and stiffness of the rail thus [5]:
(8)
\[\begin{equation} f_N \quad = \quad \frac{\pi}{2} \sqrt {\frac{EI}{m S^{4}}} \end{equation}\]where \(m\) is the mass per unit length of rail, \(E\) is Young’s modulus for the rail material, and \(I\) is the moment of inertia of the rail cross-section. Hence for a UIC60 rail with \(E = 210\) GPa, \(I = 3.055 \times 10^{-5}\) m4, \(m = 60.34\) kg/m and \(S = 0.65\) m, this comes to 1212 Hz, which is close to the note Eb6 on a piano, a little over two octaves above middle C. In practice this particular mode of vibration plays a minor role among many others that together produce a mixture of frequencies in concert with other track components such as the sleepers [34] [38].
Figure 10
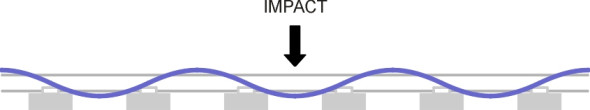
Of course each bar on a xylophone is physically separate so that when you strike it, only the one bar resonates. But a rail is continuous, and successive segments are connected to one another, so that when a train wheel impacts any given segment, its neighbours vibrate too (figure 10). The result is that vibrations propagate along the rail and noise is radiated over a wide area, damped down to some degree by the rail fastenings, sleepers and ballast. But the damping is less effective when the rails are bedded on a concrete slab, where the fastenings are ‘softer’ and the rail can vibrate over a longer length [35]. And the frequency depends on speed, so a slow- moving vehicle causes the track to oscillate at a relatively low frequency. In the case of a freight train, this is typically below 10 Hz, which is perceived as vibration rather than noise. It can be felt in buildings over 100 m from the track [36].
Damping
Broadly, there are three ways to reduce track noise radiated to the surrounding environment. The first is to ensure that the wheel and rail surfaces are smooth. The second is to build in some form of mechanical damping such as resilient rail pads. The third is to make a barrier of some kind between the track and its surroundings. Let’s look at each of these in turn.
To begin with, a rough rail surface produces more noise, and as we saw earlier in Section R1605, a corrugated surface is particularly noisy. It helps if the rail surface is as smooth as possible when it is first installed - experiments carried out by British Rail at Rugby showed that corrugations developed less quickly on rails whose surface was first treated to remove the tiny irregularities associated with the manufacturing process [15], that along with uneven rail welds tend to stimulate the wheel and rail into resonance.
Measures in the second category involve tinkering with the structure of the system itself. Some are based on the principle of a resilient material in the form of pads or mats that at some level isolate the rail from the ground. Resilient pads between the rail and sleeper reduce the stiffness of the rail structure as presented to the wheel and thereby inhibit the growth of corrugations [14] [18], but once corrugations have begun, the only remedy is to grind the rails. This was pioneered in Germany as a routine maintenance procedure [16], although it’s not as good as a new rail because the impact of a wheel on a corrugation peak produces local yielding together with residual stresses that are locked into the material below the surface and persist after the corrugations themselves are removed. These can trigger re-growth after treatment [17]. Bearing pads also reduce the level of energy injected into the sleepers. In this way, ground vibration caused by the UK Channel Tunnel Rail Link was reduced by an estimated 3 - 5 dB [2]. However, if the pads are too soft, the wave travels further along the rail, and therefore a compromise may be necessary, especially in the case of a concrete slab track, where softer rail fasteners are needed to reduce the impact forces between wheel and rail. The result is to increase the noise level by 2 - 4 dBA [35]. An ingenious way round this - at least in principle - is to space the rail supports at irregular intervals, which interrupts the natural mode of vibration of the rail. This approach recalls the irregular spacing of the cooling fan blades in a passenger car, which reduces the noise generated within the engine compartment. Another form of damping is provided by the tuned absorber, in this case blocks of steel or continuous strips of steel glued to the side of the rail. Experiments have shown that they can reduce noise by around 6 dBA, and a brief description appears in [34]. The problem is that unlike other noise emitters such as a motor car engine, a steel rail propagates energy away from the source. This energy can’t be intercepted by a single damping device at just one location; it has to be applied over the whole length of the track [32].
The third option is to prevent the noise from escaping into the surroundings. In fact the most effective measure is to put the railway in a cutting [29], and failing that, to install a barrier by the side of track [19]. Although a conventional barrier can reduce noise emissions by about 10 dBA [31], its performance depends on the geometry. Most barriers work by reflecting noise back towards the source, and the sides of a train can reflect the noise once more, this time over the barrier crest (figure 11). Barriers that actually absorb noise have been installed along viaducts on the Channel Tunnel Rail Link in the UK. They are placed quite close to the track so the height and weight can be reduced in comparison with conventional designs [1].
Figure 11
Ground waves
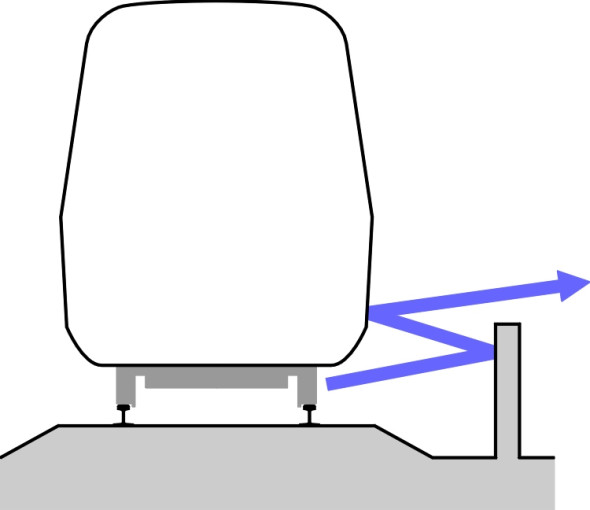
It is easy to imagine ground-borne vibrations as a kind of noise: waves travelling through the ground rather than through the air. When generated by a railway they can make buildings shake or tremble, and although the buildings are rarely damaged, the experience is not a pleasant one for the occupants. It is usual therefore to shield nearby buildings with trenches and walls [33]. But like air waves, ground-borne waves travel at a finite speed. This affects the motion of the train itself, and it is the effect on the train that we shall take up here. We’ve already seen that each axle produces in the track a shallow trough or depression. When the wheel is stationary, the trough is stationary too, centred underneath the point of contact. When the train starts to move, the trough responds by moving with it. But the response is not instantaneous. Track deflection is conveyed as a wave radiating away from the point of contact, and carrying energy like a sea wave excited during a storm. The disturbance needs time to propagate along the track and the underlying soil, and because there is a delay, the trough lags slightly behind the wheel. The result is that the wheel always seems to be climbing uphill, so that a horizontal component of its reaction with the rail adds to the resistance that the engine must overcome to keep the train rolling at constant speed (figure 12). Under normal conditions it doesn’t add very much, but as we shall later, this is not always the case.
Figure 12
Wave characteristics
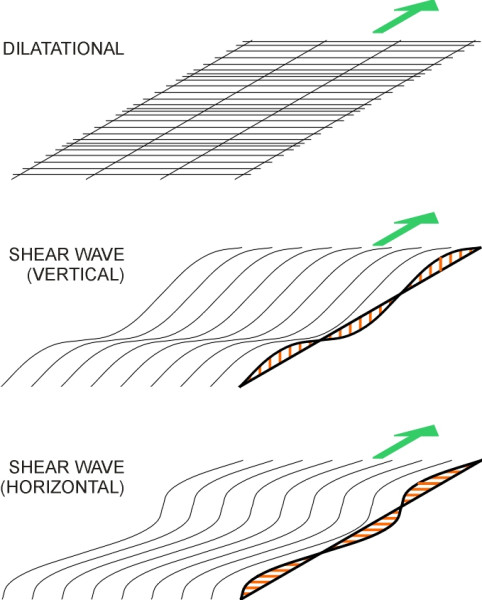
Ground-borne waves move in a distinctive way. They are made up of two components (figure 13): dilatational waves and shear waves. The dilatational wave is a pressure wave or ‘P-wave’: it alternately squeezes and stretches the ground in the direction of motion, in much the same way as the air is squeezed and relaxed in a sound wave. The second component consists of shear waves or ‘SV waves’ (the V stands for vertical), which shake the ground up and down at right angles to the direction of motion. The P-wave travels several times faster than the SV wave. There are also SH waves in which the ground moves horizontally from side-to-side at right-angles to the direction of motion, but these are much less intensive. Sooner or later, hysteresis in either the track or the ground will damp down the waves: stones and other material rub against one another, and energy is lost irretrievably in the form of heat (Section G2016). This heat loss drains energy from the vehicle, which is another way of explaining the element of rolling resistance we referred to earlier.
Figure 13
In reality, the behaviour of ground waves is quite complicated, not least because they interact with waves in the track itself, which acts as a springy structure that flexes up and down in its own characteristic way. The ‘critical track velocity’ depends on both, and a brief resume can be found in [37]. What matters here is that when a train approaches the critical track velocity the vibration can reach dangerously high levels.
Superseismic trains
Waves travel through the ground at a speed that varies according to the nature of the rock or soil through which they are travelling. In harder materials it is often around 240 km/h, but it falls to 150 km/h (42 m/s) in soft, peaty soil [20]. When a train’s speed approaches this critical velocity, the train becomes superseismic [25]. It catches up with its own waves, which pile up underneath the leading bogie and grow rapidly in size rather like the bow wave of a ship. And as with a ship, wave-like oscillations trail behind [23]. Simulation studies indicate that should a train actually exceed the critical speed like an aircraft as it passes through the sound barrier, the wave amplitude should decrease, but no-one has so far dared to try it. Waves of this kind can theoretically derail the train, and they represent greatly increased resistance, drawing energy from the power unit. To make matters worse, an embankment can act as a wave guide, reducing the effect on the surrounding environment but increasing the amplitude of deflections in the track.
The first documented case of near-superseismic rail deflections arose at Ledsgård on the newly-constructed Swedish west coast line built on soft soil, which was opened in 1997. It was designed to operate with the X2000 tilting train. At low speeds, the train rode with each axle close to the bottom of its trough, with track deflections of the order of 5 mm that were carried along with the wheels [22]. But at a speed of 185 km/h, each axle climbed to approximately half way between the trough and the crest, and track vibrations of around 20 - 25 mm were recorded. It seems that the train was approaching critical track velocity, which has since been estimated at around 235 km/h [24]. The operators were forced to impose a temporary 70 km/h speed restriction. In the light of experience gained, more recent projects have been designed with a stiffened track structure. For example, a 7 km section of the Channel Tunnel Rail Link crossing the West London marshes is supported on concrete piles designed to limit track deflections to 5 mm at a train speed of 270 km/h [4].
Conclusion
Over the last hundred years one can see a fundamental change in the way railway tracks are built. Until about 1960, the first consideration was resilience, so tracks were laid on ballast that provided a springy foundation. This minimised the damage arising from the shock loads that occurred whenever a train wheel jolted over any roughness in the rail surface or over a gap in the rail. As long as the rails were kept the right distance apart, and didn’t break, the train would not be in danger. But modern trains run at higher speeds and to avoid derailment, we no longer want the rail to move up or down by more than a millimetre or so. Logic dictates a more stable foundation - the concrete slab. To make this work, the engineer must align the rail accurately and ensure that the surface is kept smooth, so that the track injects fewer impacts into the wheel, and vice versa. The high-speed railway has become a high-precision railway with millimetre tolerances that are an order of magnitude smaller than the nineteenth century railway engineer would have thought possible.
Appendix: Strengthening the track
Two priorities are high on the railway engineer’s list: (a) reducing the stresses in the rails so they don’t break, and (b) reducing deflections in the track so the train doesn’t come off the rails. Both require the track in some sense to be made more robust, and there are two possible ways of doing this. The first is to increase the track bed stiffness, embodied in the parameter \(k\). The second is to increase the rail stiffness, embodied in the two parameters \(E\) and \(I\). Using the Winkler beam model, in this Appendix we aim to work out the sensitivity of rail stress and track deflection to each of these parameters in turn, as a basis for judging which of the alternatives is likely to have the most effect.
A sensitivity measure
A good way to judge the sensitivity of a target variable to a change in any one of the variables on which its value depends is to work out the ratio \(\rho \;\) of a proportional change in the one to a proportional change in the other, assuming that both changes are small. Suppose that the target or ‘output’ variable is \(Y\) and the input variable, whose effect we are trying to judge, is \(X\). Imagine that \(X\) changes by a small amount \(\delta X\); we can express this as a proportional change \(\delta X / X\). Suppose this leads to small change \(\delta Y\) in the target variable \(Y\). The proportional change in the target is then \(\delta Y / Y\). The ratio \(\rho\) of the two is given by
(9)
\[\begin{equation} \rho \quad = \quad \frac{X}{\delta X} . \frac{\delta Y}{Y} \end{equation}\]which in the limit as \(\delta X\) and \(\delta Y\) both tend to zero becomes
(10)
\[\begin{equation} \rho \quad = \quad \frac{X}{Y} . \frac{dY}{dX} \end{equation}\]Now, if \(X\) and \(Y\) are connected by a power relationship, this expression for \(\rho\) takes on a simple form. If
(11)
\[\begin{equation} Y \quad = \quad C X^p \end{equation}\]where the exponent \(p\) is a constant, then by differentiating both sides with respect to \(X\) we get
(12)
\[\begin{equation} \frac{dY}{dX} \quad = \quad p C X^{p - 1} \end{equation}\]We can now substitute \(C X^{p}\) for \(Y\) and \(p C X^{p-1}\) for \(dY/dX\) in equation 10 to get
(13)
\[\begin{equation} \rho \quad = \quad \frac{X}{CX^p} . p C X^{p-1} \end{equation}\]and most of the terms on the terms on the right-hand side cancel out to leave
(14)
\[\begin{equation} \rho \quad = \quad p \end{equation}\]Hence, a small change in \(X\) produces a change in \(Y\) that is \(p\) times as great, both changes being expressed in proportional terms. In everyday language, a 1% change in \(X\) produces a \(p\%\) change in \(Y\).
Stiffening the track bed
We can now get to grips with the problem of strengthening a railway track. We’ll start by looking at the effect of varying the track bed stiffness parameter \(k\) on track deflection \(w_{\max}\) while keeping \(E\) and \(I\) fixed. If we substitute the right hand side of equation 2 for \(L\) in equation 4, we get
(15)
\[\begin{equation} w_{\max} \quad = \quad \frac{1}{2 \sqrt 2} P (EI)^{- 1/4} k^{-3/4} \end{equation}\]This can be written in the form
(16)
\[\begin{equation} w_{\max } \quad = \quad C_{1} k^{- 3/4} \end{equation}\]where \(C_1\) is a (positive) constant. This is a power relationship of the same form as equation 11, and it follows that \(\rho\) is -3/4, so a one percent rise in \(k\) produces approximately a 0.75% reduction in deflection.
A similar line of reasoning can be applied to the effect of \(k\) on the bending moment \(M\). The value of the bending moment is given by equation 7. The negative sign on the right hand side is purely a convention; what we are interested in is the numerical magnitude, and therefore we’ll work in terms of the absolute value of \(M_{\min}\), which we’ll denote here by \(M_{a}\) thus:
(17)
\[\begin{equation} M_a \quad = \quad \left| M_{\min} \right| \quad = \quad \frac{1}{4} P L \end{equation}\]If we substitute the right hand side of equation 2 for \(L\) in this equation, we get
(18)
\[\begin{equation} M_a \quad = \quad \frac{1}{2 \sqrt 2} P \left( E I \right)^{1/4} k^{ - 1/4} \end{equation}\]This can be written in the form
(19)
\[\begin{equation} M_a \quad = \quad C_2 k^{-1/4} \end{equation}\]where \(C_2\) is a (positive) constant. Hence \(\rho\) = -1/4. Since for any given rail cross-section the maximum stress due to bending is proportional to \(M_a\), it follows that a one percent increase in \(k\) reduces the maximum stress in the rail by only 0.25%. This seems a relatively meagre gain.
Stiffening the rail
Now let’s see what can be gained by stiffening the rail instead. The rail stiffness is embodied in the two parameters \(E\) and \(I\), respectively Young’s modulus for steel, and the moment of inertia of the rail cross-section. Let’s deal with \(E\) first. To find how it affects deflection, we write equation 15 in the form
(20)
\[\begin{equation} w_{\max} \quad = \quad \frac{1}{2\sqrt 2} P (EI)^{- 1/4} k^{- 3/4} \quad = \quad C_{3} E^{- 1/4} \end{equation}\]and the ratio \(\rho\) is now -1/4, so that a one percent increase in Young’s modulus yields only a 0.25% reduction in deflection. To find its effect on the bending moment, we write equation 18 in the form
(21)
\[\begin{equation} M_a \quad = \quad \frac{1}{\sqrt 2} P \left( EI \right)^{1/4} k^{- 1/4} \quad = \quad C_{4} E^{1/4} \end{equation}\]Therefore \(\rho = 1/4\), and a one percent increase in Young’s modulus doesn’t reduce the bending moment at all, but rather causes it to increase by 0.25%, and the maximum stress on the rail cross-section will increase by 0.25% as well. This is not quite the paradox it seems, because we are dealing with a composite structure in which the rail and the track bed interact. A stiffer rail doesn’t flex so easily and therefore spreads the load along a greater length of track bed. In structural terms, it is working harder than would a more flexible rail.
It remains to look at the parameter \(I\). Its effect on deflections can be judged immediately from equation 15, because the parameter \(I\) plays the same role in this equation as the parameter \(E\). In other words, a one percent increase in the moment of inertia of the rail cross-section yields only a 0.25% decrease in track deflection. But we can’t apply the same trick to determine its effect on rail stress, because the assumption that we relied on earlier, that the maximum stress in the rail is proportional to the bending moment, no longer holds: the parameter \(I\) is a geometrical property of the rail cross-section, and if you change the cross-section the relationship between maximum stress and bending moment changes too.
According to the assumptions made in classical bending theory, the stress within the cross-section of a beam subjected to a bending moment \(M\) varies linearly from the upper surface to the lower surface, passing through zero at the neutral axis. Its value \(\sigma\) at any point P within the cross-section is given by
(22)
\[\begin{equation} \sigma \quad = \quad \frac{Mz}{I} \end{equation}\]where \(z\) is the distance measured vertically from the neutral axis to P, which for the sake of argument we’ll take here as positive downwards. Hence there are two peaks in the stress, one at the upper surface of the rail and one at the foot (see figure 4). In general terms we can say that the maximum absolute stress \(\sigma_a\) is proportional to \(M\) and to the rail height \(h\), and inversely proportional to \(I\) thus:
(23)
\[\begin{equation} \sigma _a \quad = \quad \text{constant} \times \frac{Mh}{I} \end{equation}\]Let’s assume that when we substitute a stronger rail, its cross-section will have the same shape with all dimensions scaled up in the same proportion, which is approximately the case in practice. The moment of inertia \(I\) is defined as the sum of each small area of the cross-section multiplied by the square of its vertical distance from the neutral axis, and for any given shape of cross-section scaled up or down in size is proportional to \(h^{4}\). Conversely, \(h\) must be proportional to \(I^{1/4}\). Also from equation 18, \(M_{a}\) is proportional to \(I^{1/4}\). It follows from equation 23 that
(24)
\[\begin{equation} \sigma _a \quad = \quad C_{5} \frac{I^{1/4} I^{1/4}}{I} \quad = \quad C_{5} I^{-1/2} \end{equation}\]where \(C_{5}\) is a constant. Hence a one percent increase in \(I\) reduces the maximum stress in the rail by about 0.5%.
But knowing the effect of changing the parameter \(I\) doesn’t help very much. The railway engineer classifies alternative rail stock in terms of weight, which is proportional to the cross-sectional area. If the height of the rail is \(h\), the area \(A\) will be proportional to \(h^{2}\). Thus \(I\) is proportional to \(A^{2}\), and in equation 15 we can replace the quantity \(I\) by \(K A^{2}\) where \(K\) is a constant. We get
(25)
\[\begin{equation} w_{\max} \quad = \quad \frac{1}{2 \sqrt 2} P E^{-1/4} \left( K A^2 \right)^{- 1/4} k^{- 3/4} \end{equation}\]and if we hold \(P\), \(E\) and \(k\) fixed this can be written
(26)
\[\begin{equation} w_{\max} \quad = \quad {C_6} A^{- 1/2} \end{equation}\]It follows that a one percent increase in cross-sectional area yields a half percent decrease in deflection. Beefing up the rail has a modest effect. But what about the stresses? If we substitute \(KA^{2}\) for \(I\) in equation 24 we get:
(27)
\[\begin{equation} \sigma_a \quad = \quad C_{7} A^{- 1} \end{equation}\]where \(C_{7}\) is a constant. So a one percent increase in cross-sectional area yields a one percent decrease in stress, which puts the option of strengthening the rail in a more favourable light.
Figure 14
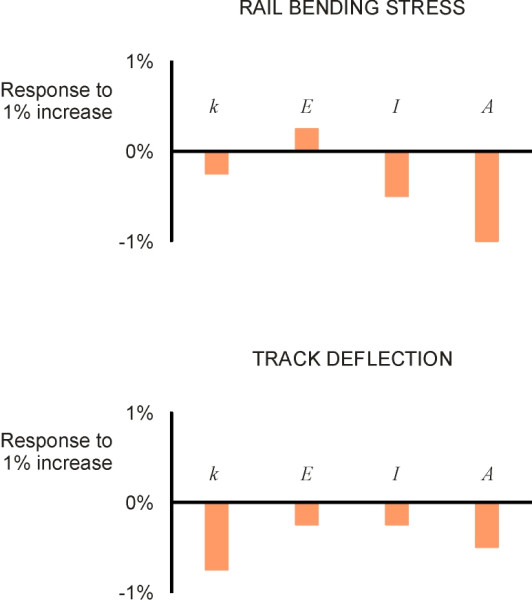
All these results are summarised in figure 14, which shows that if we consider a given percentage reduction in deflection as the target, and a percentage increase in one or other of the parameters \(k\), \(E\), \(I\) or \(A\) as the means of achieving it, then stiffening the track bed, i.e., increasing the value of the parameter \(k\), is the most effective solution. (It may not be the cheapest.) On the other hand if we consider a given percentage reduction in the rail stress as the target, then subject to the proviso that all the dimensions are scaled up in the same ratio, increasing the weight of the rail is the most effective solution.