G.0119
Rolling resistance
On a cold winter’s morning in the 1950s, it was not unusual for everyone in our household to turn out and give the family car a push start so that Father could get to work on time. It took a great deal of effort, and we were all relieved when the engine fired and the car pulled away with a jerk. Cars are more reliable today, but when they break down they are no easier to push than they were 50 years ago. Where does all that resistance come from?
The rolling resistance of a vehicle is usually taken to be the sum of all the forces acting through the wheels and axles that oppose its motion. But the value is less than you might think, around 15 kilograms for a family saloon at walking speed, which amounts to less than 2% of the vehicle’s weight [1] [5]. And once the tyres are warmed up and the car is rolling, on a level surface it can be kept rolling with one hand. The problem is not friction, but inertia. A great deal of kinetic energy must be accumulated to raise the car’s speed from zero to 12 km/h, say. To put it the other way around, at 12 km/h, the energy contained in a moving car can do serious damage. You wouldn’t want to stand in its way.
Where rolling resistance comes from
Nevertheless, rolling resistance accounts for a significant proportion of the total fuel consumed by cars and trains, and worthwhile reductions are still possible. To see how, one must understand that rolling resistance arises from several different processes that can be grouped and classified in different ways, and railway engineers and automobile engineers use different terminology to describe them. Table 1 shows how they might be fitted within a common framework. Each row of the table corresponds to a single process, with the equivalents for pneumatic tyres and steel wheels placed side by side. The grouping draws heavily on [6] and [11].
| \(\) | Category | ROAD | RAIL | Dependence on speed |
| A1 | Wheel/bearing friction | \(\) | Hot boxes | Weak |
| A2 | Wheel tread deformation | Flexing of tyre walls and tread | Deformation of tread within contact area | Weak |
| A3 | Local track deformation under contact patch | Negligible | Dent in the rail surface moves along with the wheel | Weak |
| B1 | Scrub and creep | Scrub and creep of tread along road surface | Creep and grinding losses arising from conicity, hunting, wheel and track profile wear, incorrect wheelset alignment | \(V\) |
| B2 | Suspension jounce and rebound | Track roughness converts horizontal to vertical momentum | Percussive impacts on rail joints | \(V\) |
| C1 | Aerodynamic drag acting on the wheels | Turbulence | Turbulence | \(V^2\) |
| C2 | Wheel noise | Tyre noise | Resonance within wheel disk | \(V^2\) |
| X | Large-scale track deformation | Negligible | Rayleigh waves | Non-linear |
Rolling resistance and speed
The rows in table 1 are arranged in a particular order, to match the terms of a semi-empirical formula used in railway operations. Known as the ‘Davis formula’, it was originally put forward during the 1920s, and still used today albeit with modified values for the coefficients:
(1)
\[\begin{equation} S = A + BV + CV^2 \end{equation}\]where \(S\) is train resistance expressed in force units per tonne of rolling stock, \(V\) is the speed of the vehicle, and \(A\), \(B\) and \(C\) are constants. The constant \(A\) represents all processes whose contribution to rolling resistance doesn’t change significantly with speed. The constant \(B\) represents processes whose effects vary linearly with speed. Finally, the constant \(C\) represents processes whose effect increases quadratically, with the square of speed. The rows of the table are arranged to reflect this grouping, except that there is an odd row at the bottom that, as will be explained elsewhere in the section on rail rolling resistance, doesn’t fit neatly into any of the three main groups.
Although not designed to do so, the framework seems to describe road vehicles nearly as well as rail vehicles. Most of the rolling resistance formulae that have been proposed over the years for cars and trucks have two terms: a constant term, and a term that is proportional to speed \(V\). In addition, some (but not all) have a quadratic terms as well. For normal road conditions, the quadratic term is usually ignored, and the rolling resistance of a car tyre, expressed as a proportion of the load carried by the wheel, can be taken as around 0.010 rising linearly to around 0.015 at 50 miles per hour (81 km/h) [7]. Incidentally, it increases on curves, so that a driver can expect to ‘scrub off’ speed while cornering.
But for cars and trucks moving in a straight line on a hard, flat road surface, only items A2 and B1 really matter. In fact, internal hysteresis in the tyre walls and tread accounts for about 90% of the total [14]. Scrubbing accounts for a further 8%, and aerodynamic drag on the wheel surface 2% [3] [9]. All these phenomena are quite complex and we shall deal with them more fully elsewhere: the two key items, flexing and scrubbing, are covered in section G1619, and bearing friction in G1615.
In the case of railway vehicles, consistent data for the individual components of rolling resistance are hard to find, not least because it is difficult to measure individual components when a train is actually moving along the track. There are good theoretical models for the deformation losses A2 and A3 that occur within the contact patch, but they account for only a small proportion of the total. They are often swamped by the effects of creep, especially when the wheel and track are worn (row B1 in table 1), and when you include the the impacts that occur from time to time when the wheel flange collides with the inside edge of the rail, the value comes to around 0.002 (see for example [8]).
Bumps
Most of the processes that contribute to rolling resistance are ‘steady-state’ processes that drain energy at a constant rate while the vehicle is moving at constant speed. But item B2 consists of a series of pulses whose frequency and size reflect bumps and hollows in the running surface. Although intermittent in nature, bumps nevertheless convert horizontal momentum into vertical momentum whose energy is dissipated as heat within the dampers, which is why the rolling resistance almost doubles on a worn-out road. Based on an account in [13] we can explain what happens with the aid of a simple mind picture.
Figure 1

Imagine a vehicle travelling over a step as shown in figure 1. For simplicity, we picture the vehicle’s mass \(M\) acting as a single load concentrated at the centreline of a single wheel (this will not materially affect our conclusions). Suppose the wheel and the ground surface are perfectly elastic, and no kinetic energy is dissipated during the impact. Before impact, if its speed is \(V\), the momentum of the vehicle will be \(MV\) acting left to right in figure 1. On impact, the mass will be deflected upwards. It now has a vertical component \(MVsin\theta\), while the horizontal component falls to \(MVcos\theta\). The vehicle’s horizontal speed is reduced, and if the collision is not perfectly elastic, energy is lost and the horizontal component of speed is reduced further still.
So what happens next? The wheel becomes a projectile, and executes a short hop before returning to land on the road surface downstream. When it lands, it does not recover any forward momentum, but if the collisions are elastic, it continues to bounce up and down for the remainder of the journey figure 2. If they are not, the kinetic energy will dissipate and the bouncing will fizzle out. Either way, the speed loss is permanent.
Figure 2

This means that if we want to save energy we must reduce the initial jolt. Notice that the angle of deflection depends on the wheel radius: the smaller the radius, the more horizontal momentum is lost. In particular, if the wheel radius in figure 1 were less than the height of the step, it would come to a halt (or bounce back in the opposite direction). Hence the easiest way to improve matters is to increase the size of the wheel. A large wheel reduces rolling resistance on rough roads.
Figure 3

On railways, the rail surface is relatively smooth except at the track joints. But as the wheel tread is ground away, it loses its conical shape and takes on a profile that mirrors the rail surface itself (figure 3). In this condition, two things happen. First, as will be explained in Section R1610, the steering characteristics of the axle are changed. While a conical wheelset will centre itself automatically on the track, in cases of severe wear this ability may be lost altogether, so there are more collisions of the wheel flange with the inside of the rail, in other words, more severe ‘hunting’. Each collision dissipates energy and slows the train down. Second, instead of riding on a small contact patch, the wheel is likely to scrub the rail across a large part of the profile, with possibly extended periods of two-point contact (figure 4). Different parts of the wheel are now moving at slightly different speeds relative to the rail surface, and the resulting grinding motion will remove material from both wheel and rail, dissipating energy in the process. In either case, there is more friction, and accelerated wear.
Figure 4
Suspension
Springs on a car or a railway train not only smooth the ride for passengers, they also save fuel. By insulating the body from impacts, they reduce the flow of energy that would otherwise stimulate vibration within the chassis and body panels that in turn would be dissipated as heat. Another way to visualise the process is to imagine what happens when a wheel hits a bump: the wheel rises and falls but much of the deflection is taken up by the spring. The vehicle body receives a smaller impulse and its change of direction is less abrupt than it would be if the suspension were rigid. Hence a smaller proportion of the horizontal momentum is lost, and the rolling resistance falls.
Large-scale track deformation
Finally, the last row of table 1 contains an item that doesn’t really fit into our framework at all: deformation in the running surface. By ‘deformation’ here we mean something that happens on a large physical scale. For example, each axle on a railway wagon drags along with it a depression that extends for some distance both ahead and behind the axle. When they are deformed in this way, the ballast and the ground underneath absorb some of the energy put into them and don’t give it back, so the axle experiences resistance. Another way to express the effect is to visualise the rail forming a shallow bowl, with the axle is continually trying to climb out of the bowl – effectively it is travelling uphill. This translates into a retarding force that does not act through the contact patches, but rather as a component of the vehicle’s weight acting directly to oppose motion. The bowl is not very deep: only about 1 mm [10], and the effect on rolling resistance is generally small enough to be neglected.
A similar effect occurs with roads, and again it is quite small. A well-constructed flexible pavement will deflect a maximum of 0.2 mm under a fully laden lorry, although minor roads might deflect more [4]. In general, their contribution to rolling resistance is negligible compared with tyre hysteresis arising from road surface roughness [2]. However, the picture changes when a car or lorry runs on a pliable surface. The tyre ploughs through the soft material, pushing it aside and expending energy in the process. Hence the coefficient of rolling friction rises to 0.08 on medium/hard soil and 0.3 on sand [7].
How rolling resistance is manifested
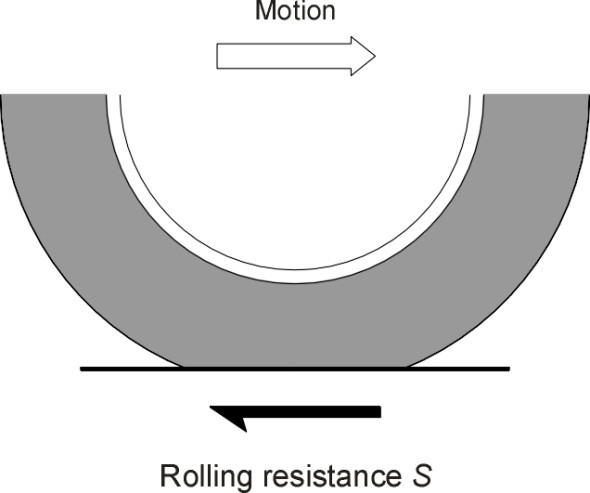
Like friction acting on the runners of a sled, rolling resistance opposes vehicle motion, and it is often treated in the same way as a classical friction. If we denote the resistance by \(S\), say, and assume that it is proportional to the normal contact force, which for a land vehicle on a level track is equal to the vehicle weight \(Mg\), then we can write:
(2)
\[\begin{equation} S = \mu_S \cdot Mg \end{equation}\]where \(\mu_S\) denotes the rolling resistance coefficient. But this raises a question. While friction can easily be visualised as a horizontal force acting on runners of a sled, it is less easy to see how an equivalent force might act on the wheels of a car or a train. Unlike runners, the wheels don’t slide along the ground: they are free to rotate. How is friction transmitted through freely-rotating wheels?
Sticky wheels
To keep matters simple, let’s examine a freely-rolling wheel (i.e., one that is not driven by the engine or being used to brake the vehicle), and look at each row of table 1 in turn. Items A1 and C1 are fairly straightforward: bearing friction and aerodynamic drag make the wheel ‘sticky’. Consider the example of a faulty bearing on a railway wagon. It will grip the axle as if to prevent it turning. Similarly, aerodynamic drag inside the wheel arch of a bus will act as a brake on the wheel surface. But these are torques, and we need to convert them into forces. Think of a toy car whose wheels are smeared with treacle. They won’t turn easily, and you will have to push the car harder than you would if the wheels were clean. What makes the wheels turn is a couple, a pair of equal and opposite forces. One of these forces is supplied by the axle at the wheel centreline, dragging the wheel along. The second is the force that opposes the vehicle’s motion, which is supplied by the road surface through the contact patch. If the resistive torque is \(T\), and the wheel radius \(r\), each of these forces must take on a value equal to the torque divided by the radius. In principle, therefore, we can depict the associated rolling resistance as a vector of magnitude\(T/r\) drawn at the level of the road surface (figure 5).
Figure 5
The shifting centre of pressure
This picture doesn’t work for the other items in the table. To isolate their effects it is necessary to set the bearing resistance and aerodynamic torque to zero. Now consider row A2 (tread deformation), and focus on what happens within the contact patch, say, of a lorry tyre that bears on the road surface with a vertical wheel load \(P\), say. When the vehicle is at rest, the distribution of normal contact pressure is symmetrical fore and aft, with the centre of pressure directly below the axle centreline. When the lorry moves, the repeated squeezing and relaxation of the rubber tyre sucks energy out of the vehicle. As each part of the tread rolls into the contact patch, it is first loaded and then unloaded, undergoing a complete stress cycle. As explained in Section G1619, since energy is lost in the process, the normal contact force between the tyre and road must be greater during the loading part of the cycle than it is during the unloading part of the cycle. But loading takes place within the leading half of the contact patch where the piece of tyre first makes contact with the road, and unloading takes place within the trailing half of the contact patch where the tread relaxes as it pulls away from the surface. Hence the distribution of pressure along the contact patch is not symmetrical (see Section C2019), and the centre of pressure moves forward. Denote the shift by \(e\) say. The normal contact force now exerts a torque \(Pe\) about the axle centreline. Since the wheel is rotating at constant speed, this torque must be balanced by an equal torque in the opposite direction. It can only arise from a horizontal shear force \(S\) through the contact patch acting in the opposite direction to motion, and this is the rolling resistance component we are looking for. By taking moments about the axle centreline, we get \(Sr = Pe\) and thence \(S\). Another way of deriving this relationship is to look at the geometry: the resultant force exerted by the road surface must pass through the axle centreline, as shown in figure 6. This overall force, which we shall call \(R\), must have a vertical component equal to the wheel load \(P\), and a horizontal component \(S\). From the associated parallelogram of forces it is obvious that \(Sr = Pe\).
Figure 6

The processes B1 (scrub) and C2 (wheel noise) in table 1 are more readily visualised as energy losses that can be directly translated into resistance forces using the fact that \(\textit{energy flow} = \textit{force} \times \textit{speed}\) (which in turn follows from \(\textit{work} = \textit{force} \times \textit{distance moved}\)). The same applies to deformation of the road surface immediately under the contact patch, each segment of which is loaded and unloaded in turn as the wheel passes over. Unless the material is perfectly elastic, the resulting loss of energy will appear as an additional component of rolling resistance (row A3 in table 1). All the above applies to railway vehicles too, which might seem surprising because the wheels and track are made of steel, which we usually think of as ‘elastic’. However, the material is not perfectly elastic, and in practice small energy losses occur within the contact patch.
In this Section we have concentrated on the resistance experienced by non-powered wheels because non-powered wheels are easier to analyse. It only remains to point out that for powered wheels the principles are the same, except that some of the components of rolling resistance such as bearing friction draw energy directly from the drive train. They slow the vehicle down, but their effects are not visible in terms of a shear force component at the contact patch.
A footnote
As a footnote, it is interesting to compare road and rail vehicles with vehicles of other kinds. How about skis? The lowest value of the coefficient of friction of skis on snow is about 0.03. A car does better. When travelling on a smooth, hard road surface, the rolling resistance coefficient lies between 0.01 and 0.02, typically around 0.015 at 50 mph [7]. Trams have a lower coefficient still (see for example [8]), and their low rolling resistance is one of the reasons often put forward in support of a new tramway scheme, although in reality trams are not much better than buses. For a heavy railway coach or freight wagon, the coefficient of rolling resistance is miniscule, around five to ten times less than a road vehicle [8]. But this is not a fair comparison. Rail vehicles are heavily built, and although the coefficient is small, the weight is large, and when the two are multiplied together to calculate the resistance force, some of the advantage disappears. Much more impressive is the MAGLEV train, which has no rolling resistance at all.